In mechanical properties of various solid materials, two of the most important concepts are that of stress and strain. It is crucial in understanding how an object behaves or reacts when you apply a force on it.
Stress and strain help in identifying the strength, deformation, and various other mechanical properties of the object. If you’re a structural engineer or are looking to deal with different objects, you got to know how much load or stress an object can withstand before it breaks, distorts, or stretches.
So in our today’s episode of explainer videos, we’re going to delve into the concept of stress strain, how they’re related to each other, and also at the end, we’ll discuss stress strain graph and Hooke’s law.
Make sure to watch till the end. I’m adding a spice of some interesting real-life examples that will blow your mind.
So let’s get started.
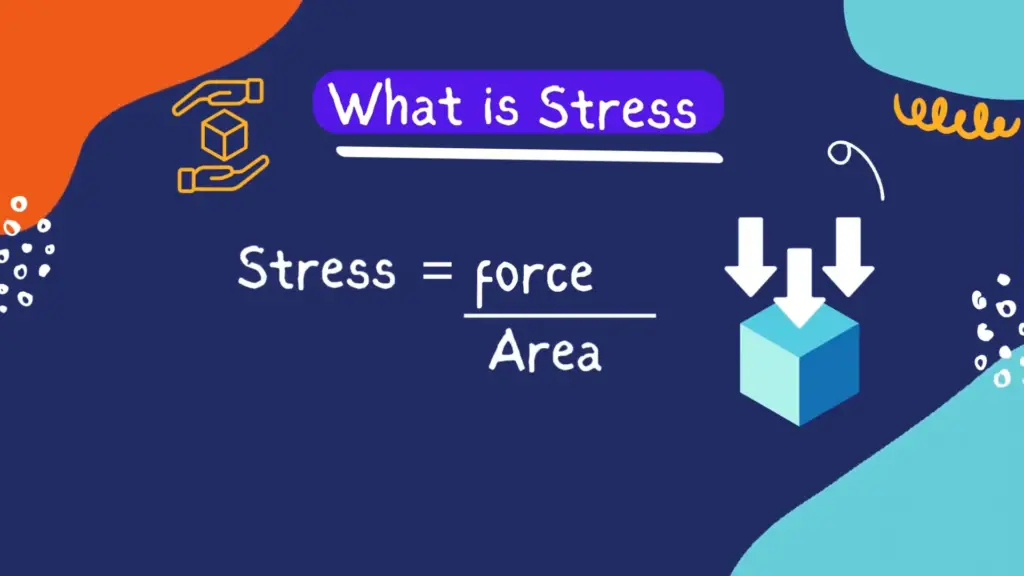
What’s the stress?
In physics or material engineering, the word stress has a different meaning than that of the medical field. Stress is basically force per unit area within materials that arises from externally applied forces or I can say it can also be due to uneven heating or permanent deformation.
Well, in other words, stress is an indication that describes and predicts elastic, plastic, and fluid behavior of any object.

What do you be doing to determine stress?
Well, pretty simple, you just take the sum of all forces acting on an object and divide that to the area of the object where force is acting and this will give you a measure of stress.
So applied stress or Sigma is equals to summation of forces divided by unit area. Stress has a unit of Newton per meter square.
Like Us on Facebook!
There are two basic types of stresses: tension and compression stresses. I’ve got another amazing explainer video for that so make sure to watch that as well.
Subscribe Us on YouTube!
You know why some materials are called brittle or some as ductile like a glass is brittle while a steel bar is ductile?
Well, that’s because glass takes less stress before failure while steel takes more stress before failure. Similarly, you can stretch a rubber band far apart but you can’t do that with a wither paper.
Yes, it will be torn. So you see different materials behave differently and to understand their behavior you got to understand the concept of strain.
So what is the strain?
Well, a strain is known as deformation in an object due to applied external force in a particular direction. For example, if you push down this spring, it will have a reduced length.
So the difference of this length in comparison to the original length will be called strain in the spring. Similarly, if you pull a rubber band, it will have increased length.

So this increased length divided by the original length will be the strain in the rubber.
So basically, we can say that strain explains the change in the shape of the object or deformation due to the load or force being applied onto it. Mathematically, we can express strain as Epsilon equals Delta L / L. Now it’s a unitless property meaning it does not have any unit. It is a dimensionless quantity.
It is because it just compares the two same characteristic of an object. There are different types of strain like tensile strain, compressive strain, shear strain, etc. We’ll discuss this in our future videos so make sure to subscribe.
Let’s now move on to the next part of this video that is about stress-strain curve. When we study solids and their mechanical properties, information regarding their elastic properties is most important.
We can learn about the elastic properties of materials by studying the stress-strain relationships under different loads. In these materials, the material stress-strain curve gives its stress-strain relationship. In a stress-strain curve, the stress and its corresponding strain values are plotted. Here’s an example of a typical stress-strain curve.
Let’s now discuss about the different regions of the stress-strain curve:
Proportional limit
It is the region in the stress-strain curve that obeys Hooke’s law. In this limit, the stress-strain ratio gives us a proportionality constant known as Young’s modulus. The point OA in the graph represents the proportional limit.
Elastic limit
It is the point in the graph up to which the material returns to its original position when the load acting on it is completely removed. Beyond this limit, the material doesn’t return to its original position and a plastic deformation starts to appear in it.
Yield point
The yield point is defined as the point at which the material starts to deform plastically. After the yield point is passed, permanent plastic deformation occurs. There are two yield points: upper yield point and lower yield point.
Ultimate stress point
It is a point that represents the maximum stress that a material can endure before failure. Beyond this point, failure occurs.
Fracture or breaking point
It is the point in the stress-strain curve at which the failure of the material takes place.
Difference between Stress and Strain
So now that you know what is stress and what is strain, let’s now see what is the difference between stress and strain.
Well, in physics, stress refers to the force that is acting per unit area of the object whereas strain depicts the ratio of the change in an object’s dimension to its original dimension.
In physical parlance, stress is equivalent to pressure and its unit is Pascal (Pa) or pounds.
On the other hand, strain signifies the ratio of change in dimension to that of the original dimension, therefore has no units of measurement. Strain, however, can be measured by strain gauges.